Gravitational Waves
Gravitational waves have recently brought much attention to the phenomenon of spacetime by pointing out that space itself is a physically ductile field property of reality. In giving this idea a bit of rational thought, one should be able to draw the conclusion that visual perspective must be a manifestation of at least some aspect of this same “spacetime”. But how…?
_____________________________
Painters Throughout History
For centuries artists have grappled with certain perplexing features of spatial perspective (see; perspective), which cause distortions and the curving of straight lines. The same phenomenon causes an exaggeration of features in portraiture known as foreshortening. Artists and photographers often employ foreshortening for creative effect and it is the same distortion found in photographs when a camera is held too close to a subject’s face, rendering an over-sized appearance to the nose or other features. To minimize the undesired aspects of this effect, artists have traditionally moved their subjects (or the picture plane) back three to five meters. But, why should the distortion be there in the first place? These distortions would be enigmatic if considered from the point of view that space is “nothing” or “a void”. If on the other hand one subscribes to the view proposed by Albert Einstein that space is a facet of spacetime or the gravitational field, then it should not be a great leap of logic to accept the view that visual perspective might be some manifestation of a transforming energy field.
Foreshortening can be seen in the preceding sketch where the glass and hand appear disproportionately large when compared to the head in the background. In the following photograph, the dog’s head appears tiny compared to a portion of its tail in the foreground (see; the black dashes for scale…both sets of dashes are identical in size). 
These phenomenon are I perceive caused by the fact that our universal world, the space included, exist within a transformational geometry that is expanding in the same manner that light expands outward into our universe. These transformations are at the root of the aforementioned artists’ quandaries and I believe it can be shown to be the cause of visual and spatial perspective, and even external reality itself.
By looking at a larger model, the above-mentioned distortions can be magnified for easier analysis. We can do this by looking at a long hallway as demonstrated in the following drawings. What we are essentially doing with the following thought experiments is measuring 3-dimensional space and then projecting it onto a 2-dimensional surface. However, something more profound is happening, which will be elaborated more succinctly in the previous section.
Some might argue that by examining 3-dimensional-space within the context of 2-dimensional-space, we are merely examining distortions of reality, not reality itself. My counter-argument would be that visual perspective (the way 3-dimensional-space appears) is itself a distortion of reality and that the foreshortening and curvatures outlined here are manifestations of the same phenomenon causing all distortions related to visual perspective. The cause; delayed transmission of images. That is to say, light having a finite velocity, cannot therefore have an instantaneous transmission time. This incorporates time into all elements of reality via an expanding matrix. The following area has been created in order to show what the issues are and to provide tools for verifying what is herein offered as a solution.
Overcoming Preconceived Ideas
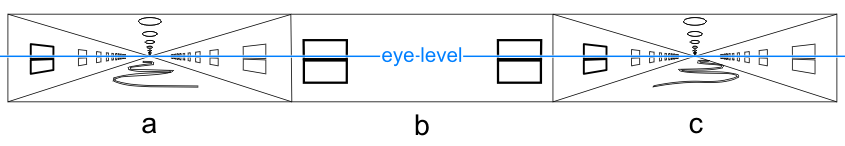
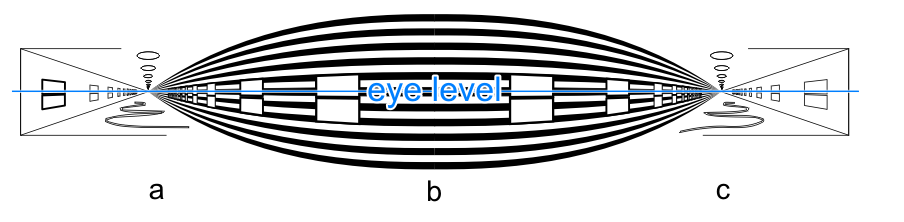
Our brains have remarkable capabilities to appease our sense of aesthetics or preconceived notions, sometimes at the expense of accuracy or truth (example see punctum caecum). This can make it difficult for most people standing in a long, straight hallway (see Fig.1 abc) to detect any curvature at the junctures of the wall with the ceiling and floor as the view sweeps past them. One needs strong peripheral vision to do so and even then, one must suppress their left cerebral hemisphere to prevent their seeing the straight lines our intuition tells us we should be seeing. How do the diagonal lines rising from the vanishing points in a and c below, meet or transition into each other as represented by b without either curving or making a jog as seen in the drawing. Common sense and logic should make one ask the question; how do the left / forward / right views transition the straight line without curving it? It should be added that this is not simply a problem of transposing the 3-dimensional curvature onto a two dimensional surface. One can perceive that the curvature actually exists in 3-dimensional space by using the minds eye to perceive what their 2-forward-looking eyes can’t. I believe if the reader follows the line of reasoning here and in the next section: Spatial Perspective and the Arrow of Time, he will come to agree that the reason for the seeming quandary is that our spatial perceptions are time-dependent and that the only way to reconcile the seeming ambiguities of the curving of straight lines, is to view our entire sense of reality as embedded within an expanding matrix.
We’ll start with the following demonstration of how straight lines can be shown to curve, which in turn will lead us to the cause of the aforementioned effects. It can be established by a thought experiment of standing sideways (facing one wall) in a single, long hallway and looking down the corridor in both directions (Fig.1.abc).
First we look left down the hallway (Fig.1.a), take a snapshot, look in front of us (Fig.1.b), take a snapshot, then look right (Fig.1.c) and take another snapshot. This is necessary for our purpose because we as humans, having two forward-looking eyes, can only see in one direction at a time. If we had compound eyes like a fly and a brain to decipher the images, we could probably see all three views at once but we don’t, so we have to settle for adjusting our position for each of the three views. It is important to remember though that the three views are in reality part of a straight, single, continuous wall. We need the three views illustrated this way because (I’m assuming that) you cannot see all three views at once with your normal vision. If however you close your eyes and imagine what is happening from viewing the ceiling and floor lines in the left view (Fig.1.a) then jump to imagining the right view (Fig.1.c) you should realize that what are straight lines can only get from your left side to your right side by curving past you. In fact, there is no way (on a 2-dimensional surface) to smoothly depict the transition from left to right without either: 1. Bringing straight-perspective-lines together at points above and below your eye-level: 2. Curving the same lines: or…3. Doing as is shown below (Fig.1.b…splicing in a parallel section to represent that area of wall in front of us). As you will see below, curving the lines is the truer interpretation.
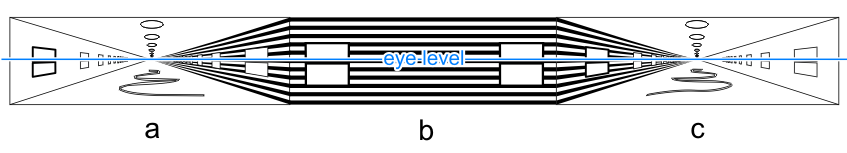
To help familiarize us with the idea of a transformational geometry, we will depict the same view as Fig.1abc, but with black lines painted on one of the two side walls as seen in Fig.2.abc.
Hopefully, if you didn’t know it before, you are starting to realize that the space surrounding us possesses some very strange qualities. The following areas are going to demonstrate the actual distances from what would be different points on the wall to your eyes. The one important thing to remember is that the distance from any point in the space around you, relative to the distance of any other point from your eye, is the time delay that light from that point would take to reach your eyes, i.e.; light leaving a point 11 units of distance from your eye would take longer to reach your eye than light from a point 10 units of distance from your eye. The longer distance means that the image is arriving from further in the past. If you contemplate this within the context of an expanding matrix, then you will understand why the curvatures appear. This section hopefully provides the tools needed to verify what is explained in more detail in the following section; (Spatial Perspective and the Arrow of Time).
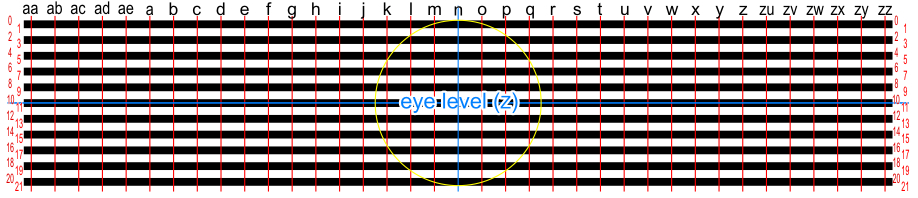
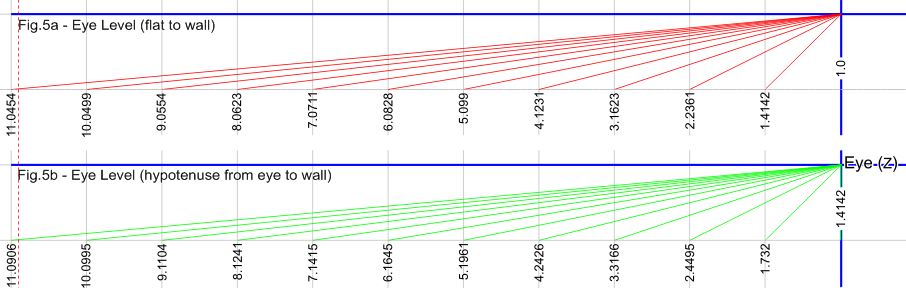
In standing in front of a section of the wall in our hallway, we will stipulate that our eye-level is half the height of the wall and we are standing that same distance from the wall. In order to create measuring points, we’ll place vertical red lines spaced about 36 cm apart intersecting the horizontal black and white bands (Fig.3). These will act as Cartesian coordinate system to help us understand what happens to a 2-dimensional space within the context of a 3-dimensional space. The coordinate axes will be the horizontal-blue, eye-level line and the vertical-blue line at position n. This means that the shortest distance to the viewer’s eye (z) would be at junction n10.5 (I must apologize for using half increments here but I wanted the bands to start and end with black thus obligating an odd number of bands).
Measurements with Triangles
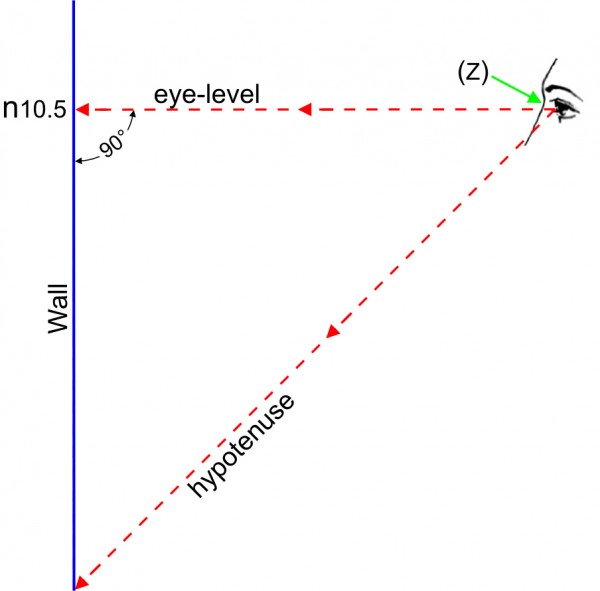
The position of the viewer’s eye will be z as seen in Fig.3, Fig.4 and 5b. All lines made from n10.5 to any point on the yellow circle in Fig.3 will be equal in length so that all triangles tracing from z to n10.5 to any point on the yellow circle will be identical in size to each other, only their orientation will differ. This particular group of triangles will all be isosceles right triangles. All others, inside or outside the yellow circle will simply be right triangles, because as seen below in Fig.4, the leg from z to the wall will always be 90°.
In Fig.5a (the uppermost drawing of Fig.5…red), we are seeing an expanded view of the wall from behind the viewer’s left side (from Fig.4) with diagonals from the closest point on the wall from the viewer’s eye (z) to coordinate points below eye-level. Each line left is the diagonal of an ever longer rectangle with its corresponding length posted below it.
From this same angle, Fig.5b (green) looks identical in length to Fig.5a but in 3-dimensional space, it is not. Whereas all the lines in Fig.5a are diagonals on the wall; all the lines in Fig.5b originate at point (z), and are represented by the hypotenuse from the viewer’s eye in Fig.4 and proceed to the same end-points as each corresponding diagonal in Fig.5a. That is why the blue vertical axis has a length of; 1 in 5a but 1.4142 in 5b. The 1 measures a single side of a square, whereas the latter figure represents the diagonal of a square from the viewer’s eye (see z in Fig.4).
What Does the Above Have to do With the Curving of Lines?
If one takes the above method of measuring a wide angle array of any points in space as seen by a viewer, for example the hallways in Figs. 1, 2 and 3, it will be seen that straight lines will curve as depicted in Fig.6 (below). But why does it happen that way? This is the subject of most of the rest of this treatise but for its direct relationship to perspective, see; Spatial Perspective and the Arrow of Time.
What is the Rate of Expansion Inferred to Here?
The rate of this expansion is the speed of light “c”, however in order to understand what is really happening, it cannot be thought of as either a speed or velocity. It is what could be referred to as a “dynamic-reaction- constant”. This is the same constant that is found in the familiar expression mc². However, within EMT it has an amplified meaning in that with visual perspective, it represents a linear expansion constant of scale for any physical object. That is to say, any object under observation, regardless of size, is expanding at this same constant, because of the way the total of all fields interact upon the “surface” of any object. This constant means; that although all objects are expanding at a tremendous rate; they maintain proportion to one another, within any given frame of reference. This constant becomes a constant of speed for light because it is measured via surface. However, by inference, it can be shown to travel either much faster or much slower than this speed. The deeper reason for this is that space is a function of time, rendering speed a deceptive gauge; for example mph = distance/time, but if distance itself is a function of time, then the equation becomes ambiguous and could be said to read: time/time. The nature of the issue can be explored in Spatial Perspective and the Arrow of Time.
Visual Perspective – Function of Distance or Time?
Visual perspective is understood as a function of spatial distance. Herein though, it is explained to be a function of time. This would in many people’s mind seem to be splitting hairs. That is because the two can often be interchanged with no differences in results. There are instances however, when they cannot be intermingled. In this treatise for example we are using drawings and scaled measurements to follow the logic, so we use terminologies for space and distance (1/d) but the reader should eventually perceive that time-based definitions (1/t) can be the only definitively accurate basis for measurements.
Relative linear size found in perspective normally follows an easily traced formula of one divided by distance (1/d) where the basic gauge or scale would be: 1/1. Because the eye of the viewer is the starting point for all observations, most if not all measurements start there with the gauge (1) being a certain size and a certain distance from the eye. On earth the horizon always appears at eye-level to the viewer, whether he be crouched low down or high up in an airplane. Likewise the vertical axis should appear straight up and down. All perspective values start and radiate from these two axes see; Eye Level and the Horizon.
The area we are considering here is 5b as it applies to the perspective parameter, which is the hypotenuse from the viewer’s eye to specific points of the grid and it is this distance that produces the relative sizes or spans between different points. That is to say, the hypotenuses represent the value (1/d) as the perspective parameter based on the gauge (1), which is the shortest distance from the viewer’s eye directly to the wall. If one were to make their own graph based on the method of building out from the two aforementioned axes, they would arrive at a drawing of the three-view similar to that seen below in Fig.6abc (when seen with smart-phones these images seem to distort excessively…to see them correctly, load them on a PC).
Readjusting our Perception
This is where the reader should sit up and pay attention: Remembering that the picture represented by Fig.6abc is a close approximation of how space actually curves around us, one can see how these resultant curvatures correspond to field lines found for example between magnets. I’m not trying to invoke magnetism here but to nudge the viewer into thinking in terms of field-transformations. In fact there is another term I would like to introduce in order to move us beyond the idea of a spatial-field to the idea of a “time-field”. The concept of a time-field is a training mechanism intended to help us perceive spacetime first as a field, then separate the spatial part of the field from the temporal part. By looking at both elements within their contexts then recombining them, it is hoped that the reader will see spatial perspective as a time–dependent mechanism.
Contemplating this may at first seem nonsensical because as an observer walks down the hallway, the effect follows him as if the observer alone is causing the effect. When this first occurred to me sometime prior to 1984, I rejected it because “cause and effect” seemed to be reversed. I had all but forgot about it except that something was happening which later initiated the tantalizing notion that qualities of space producing visual perspective may be the result of temporally-dependent energy transformations. It took several years of reflection on empty space and gravity for a conceptualization to form about the cause of these effects and what reality is at its deepest level.
Photographing the Curving of Straight Lines
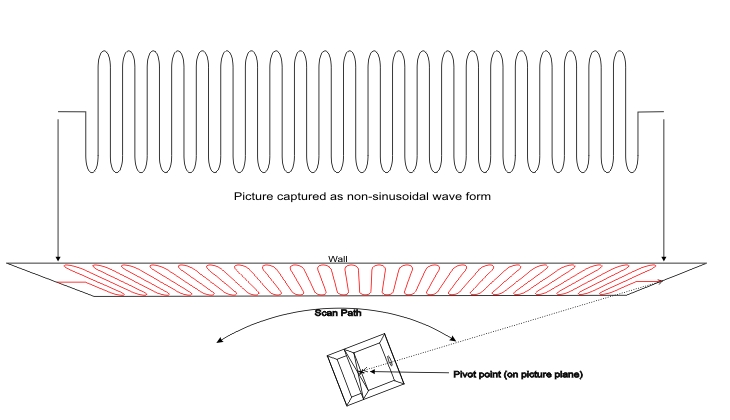
Most people have seen photographs taken with extra-wide angle lenses and are familiar with how straight lines will appear as curved lines in these photos. What many don’t realize is that most of what they perceive as distortion, really exists. Every focal length is different and I don’t know if there exists a standard optical lens that would give a perfectly accurate 180° picture but in 1993, I did suggest a camera to a manufacturer that would shoot a 360° picture anywhere. To appreciate the perfection of such a camera for this purpose, imagine a camera that takes a series of one-pixel wide adjacent, vertical lines, as it rotates up to 360° on a horizontal plane. It stitches each vertical line to the next and thus produces a perfect panoramic view that amounts to a series of non-sinusoidal lines. Fig.7 below gives a simplified view of how such a camera would work.
Such a camera would give the best possible assimilation, of all three views. In the example of our hallway as seen in Fig.6 Lines would curve most at the periphery of our vision where the view sweeps past us. The single horizon line (eye level) and vertical axis would remain straight but all other lines would warp increasingly with distance from our point of vision (Fig. 6b).
The view in Fig.6 probably scans about 240° around our periphery and a flawless scan should produce the same results as calculations using 1/d based on the hypotenuses arrived at in Fig.5b. Such a photo-scan would be much quicker than the meticulous process required to produce the same picture mathematically. This is because to be accurate using the mathematical method, one would have to account for the hypotenuse from the viewer’s eye to each individual pixel-point point seen in a given view. However, results arrived at from whichever method, should give an accurate representation of the reality.
Summary
I hope I have supplied enough information on this page to initiate the reader to the fundamental elements of visual perspective and its ambiguities. The term visual perspective has been used here as if it differs from spatial perspective but they are for the most part, the same. Instances may arise when one might feel compelled to make a differentiation. A marksman for example shooting at a distant target must treat a large distant object identical to the way he would treat a tiny object. He must also take into account forces such as wind, gravity or Coriolis. There are also geometric effects such as triangulation or parallax that can make a difference but once these are accounted for “visual perspective” still has the same meaning. For all intents and purposes, a distant object “is” a tiny object because at the moment when the light left the object, it really was comparatively that “tiny” size


This article is really interesting. I have bookmarked it.
Do you allow guest posting on your page ? I can write
hi quality posts for you. Let me know.
can you give me details?
Yes, if you can give me a specific question.
Thank you Alba. So far, I don’t have a specific blog page. That is mainly because of a lack of time. I have several more unfinished core pages on the go. I am however willing to create a page for physicists or mathematician to present their proofs or objections, providing they don’t use as proofs, ideas that are simply tautological or an arbitrary preference in the conflict between classical, quantum and relativity theory. That is of course unless it has a meaningful train of logic that might reconcile the conflicts (there’s already plenty of reasons to show why they can’t be reconciled). As an example; an astronomy instructor in England was vehemently using Newtonian mechanics as the basis for his argument, when Einstein has already proven Newtonian mechanics to be incomplete (fundamentally wrong). It would be a nonconstructive and frustrating waste of time to give platform to someone who just wants to argue a moot point.
I do however have a Twitter account: @EMT_INFO
Thanks again for your interest…